What is Conjugate Complex?
A conjugate of a complex number
is defined as
The number
and
differ only in the sign of the imaginary part. The real parts are the same.
Example
then
Representing in polar form, if
then
-
The magnitude is
-
The direction is
. Note the argument of the results must be reduced mod
to values in the range of 0 to
.
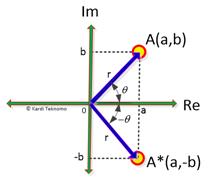
Graphically a conjugate complex is the reflection a complex number with respect to the real axis. Drawing below illustrates a complex number and its conjugate.
Complex Number Calculator
Properties
The following are useful properties of conjugate complex:
-
A conjugate complex of a conjugate return the complex number itself
-
Addition of complex conjugate
-
Subtraction of complex conjugate
-
Multiplication of complex conjugate
-
Division of complex conjugate
-
The real part of a complex number can be obtained from
-
The imaginary part of a complex number can be obtained from
-
Square absolute of a complex number
See Also : Arithmetic of Complex Number
Rate this tutorial or give your comments about this tutorial