Quadratic Growth Model

<Content | Previous | Next >

Difference equation:

Parameter is constant of second difference, and parameter is the value of x (1) when x (0) = 0

Functional shortcut:

Characteristics:

• Add amount that grows linearly with
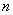
• The first differences are arithmetic growth or linear
• The second differences are constant

Try to experiment with the interactive program of Quadratic Growth Model below. Input the initial value x(0) and constant g and h then click "Find" button to find x(n) and to show the plot.

Quadratic Graph

• The shape is 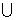for positive 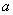and 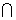for negative
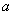
• The graph crosses the 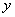-axis at (0, )
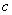• The graph is symmetry with vertical symmetrical line at
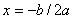
• The highest or lowest point of the graph happens at
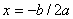
• The value is called discriminant. If the discriminant is negative, the graph has no 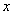intercept (does not cross to 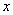-axis). If the discriminant is zero or positive, the graph has 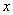intercept at

<Content | Previous | Next >

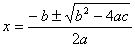
See also: Dynamical System tutorial

Rate this tutorial

This tutorial is copyrighted.

Preferable reference for this tutorial is

Teknomo, Kardi. Simple Growth Model. http:\\people.revoledu.com\kardi\ tutorial\Growth\