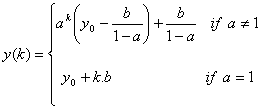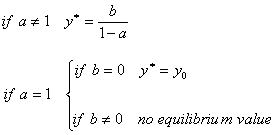<
Previous
|
Next
|
Contents
>
Affine Dynamical System
The difference equation
is called affine dynamical system. It is one of the simplest forms of linear difference equation. It has characteristic of first order linear discrete dynamical system with constant coefficient and constant input to the system. Table below shows the equation, solution, equilibrium value and stability of such system.
Difference equation |
|
|
Solution |
|
|
Equilibrium |
|
|
Stability |
Stable if and only if
Unstable if other cases (i.e.
|
Equation( 4 ) |
or
)
Notice that the solution depends on both the difference equation and the initial condition. Derivation of the solution and equilibrium are shown in previous pages .
See Also:
Numerical Example
<
Previous
|
Next
|
Contents
>
Preferable reference for this tutorial is
Teknomo, Kardi (2015) Difference Equation Tutorial. https:\\people.revoledu.com\kardi\ tutorial\DifferenceEquation\